(1-x^2)y''-2xy'+2y=0 power series 283757-(1-x^2)y''-2xy'+2y=0 power series

Answer (1 of 2) Solve ( d^2 y(x))/( dx^2) ( dy(x))/( dx) (x 2) y(x) = 0 Let y(x) = (x 2) v(x), which gives ( dy(x))/( dx) = ( dv(x))/( dx) (x 2) v(x

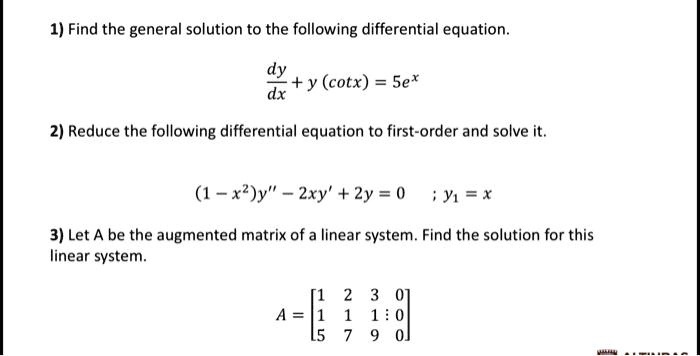
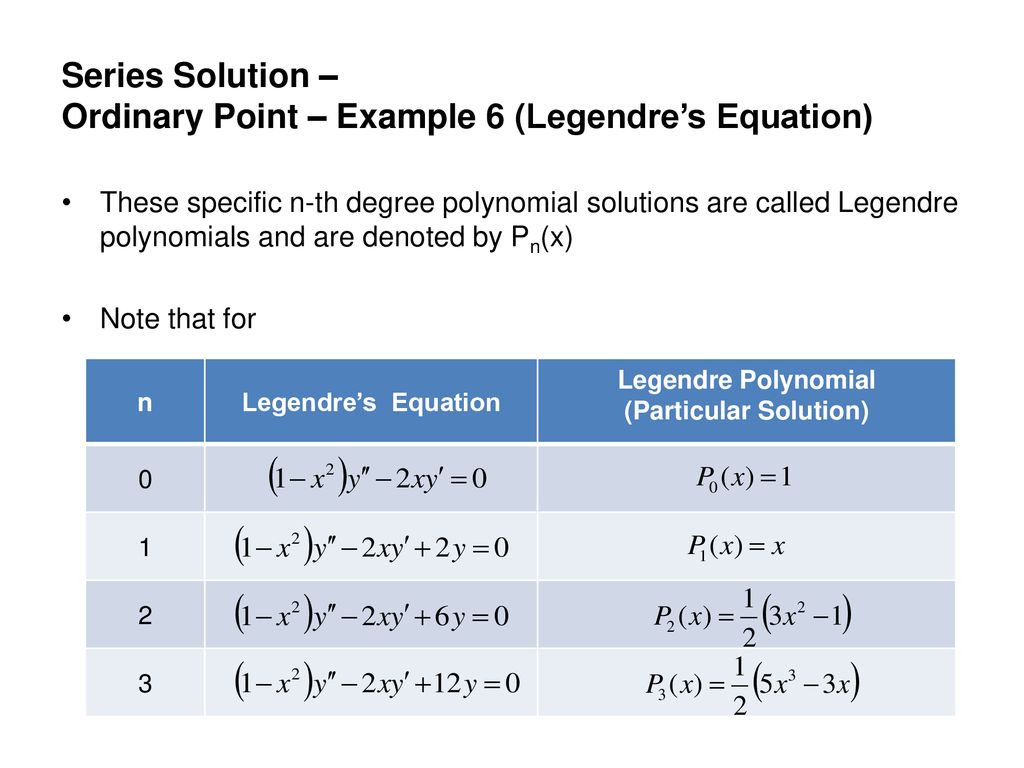
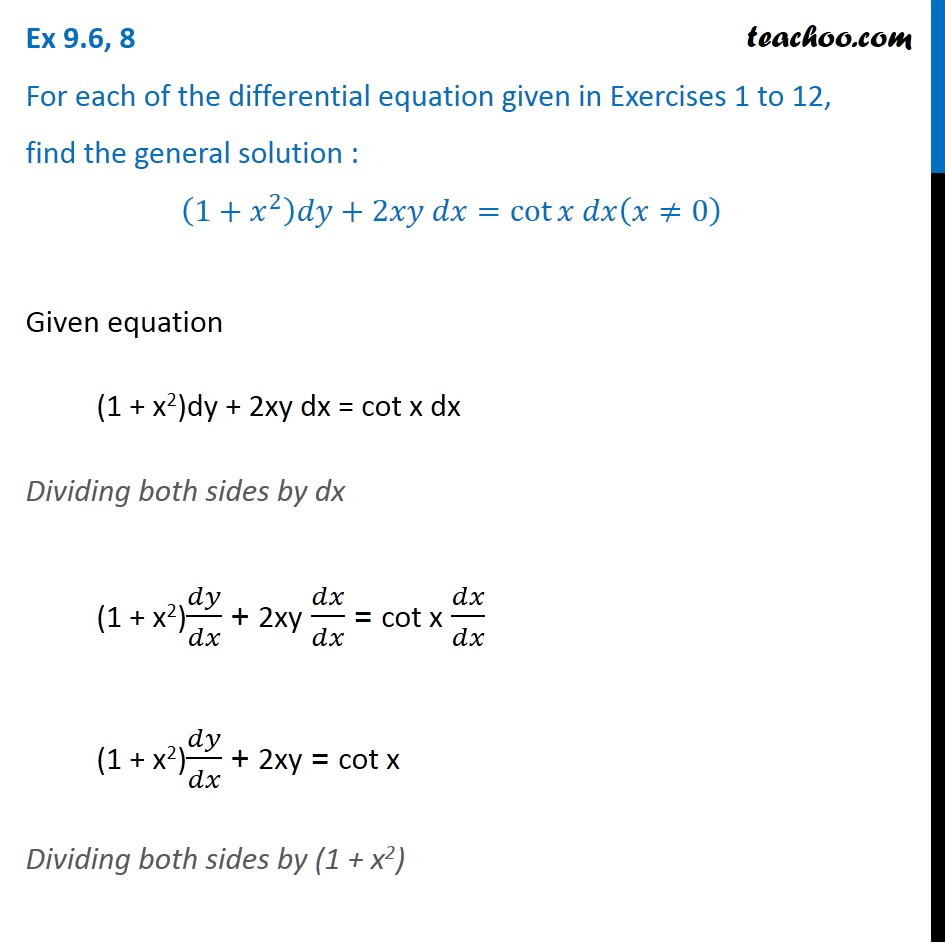
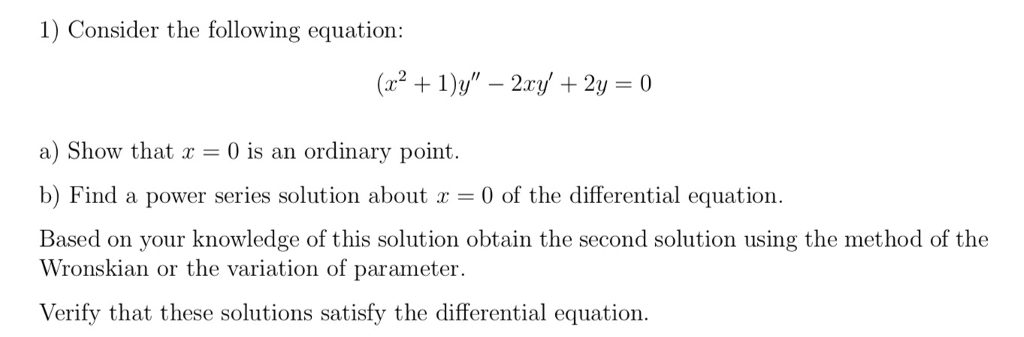
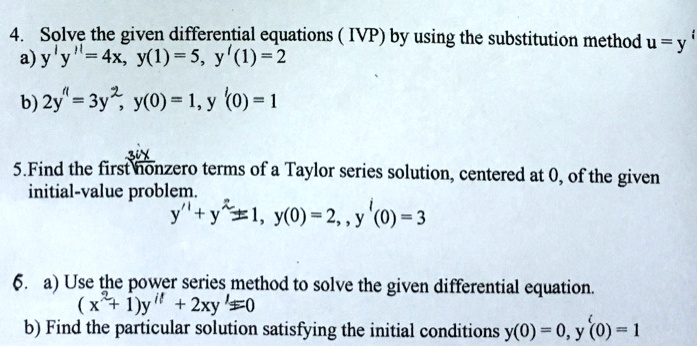
(1-x^2)y''-2xy'+2y=0 power series-(1 − x^2)y'' − 2xy' 12y = 0 (i) Consider a power series solution centered at zero and derive the recurrence relation for the coefficients (ii) We know from the general theory that there are two linearly independent solutionsFind the power series solution in powers of x show the details (1x^2)y''2xy'2y=0;

(1-x^2)y''-2xy'+2y=0 power seriesのギャラリー

各画像をクリックすると、ダウンロードまたは拡大表示できます

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「(1-x^2)y''-2xy'+2y=0 power series」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「(1-x^2)y''-2xy'+2y=0 power series」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「(1-x^2)y''-2xy'+2y=0 power series」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「(1-x^2)y''-2xy'+2y=0 power series」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「(1-x^2)y''-2xy'+2y=0 power series」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「(1-x^2)y''-2xy'+2y=0 power series」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「(1-x^2)y''-2xy'+2y=0 power series」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

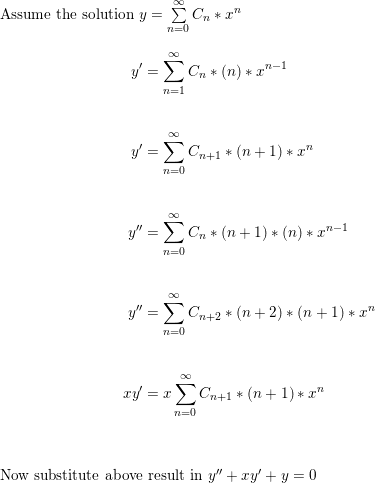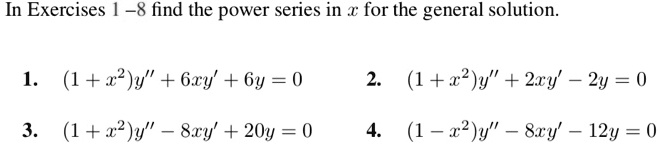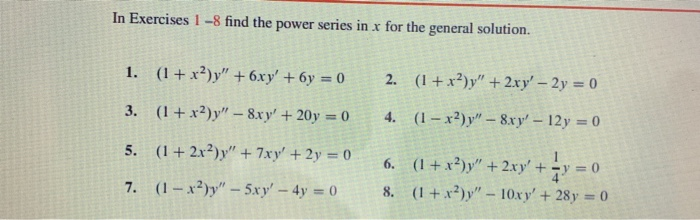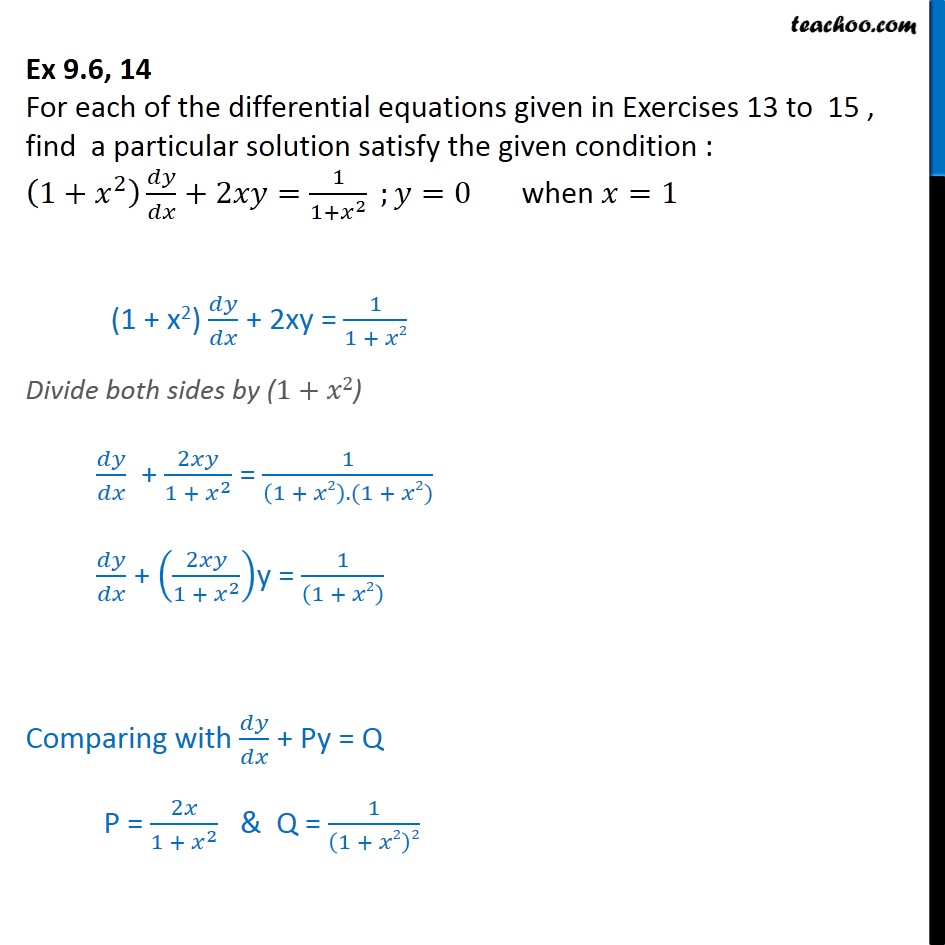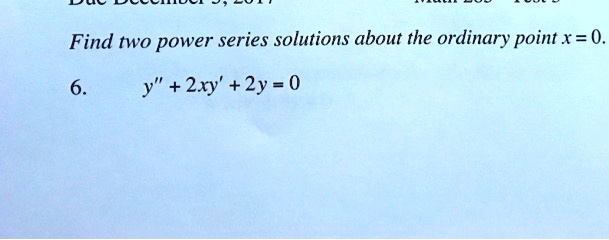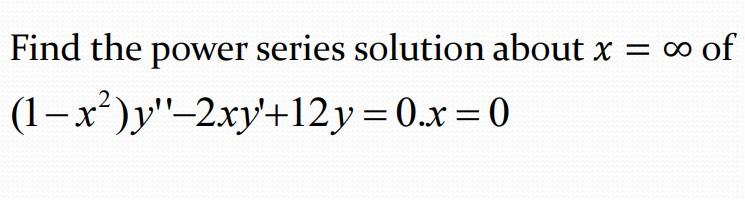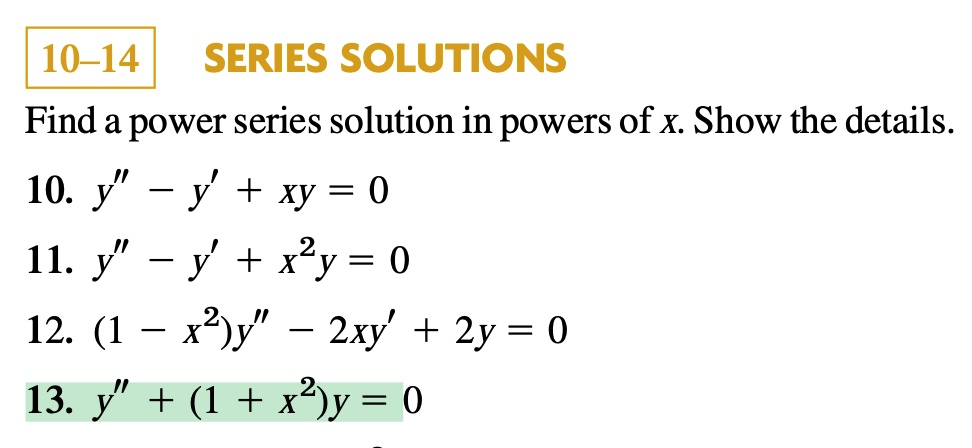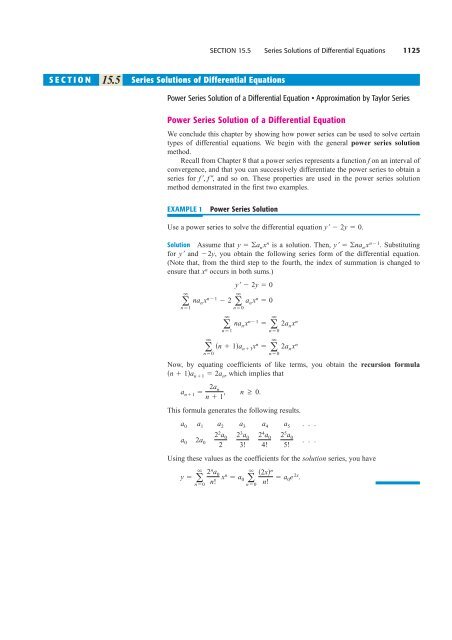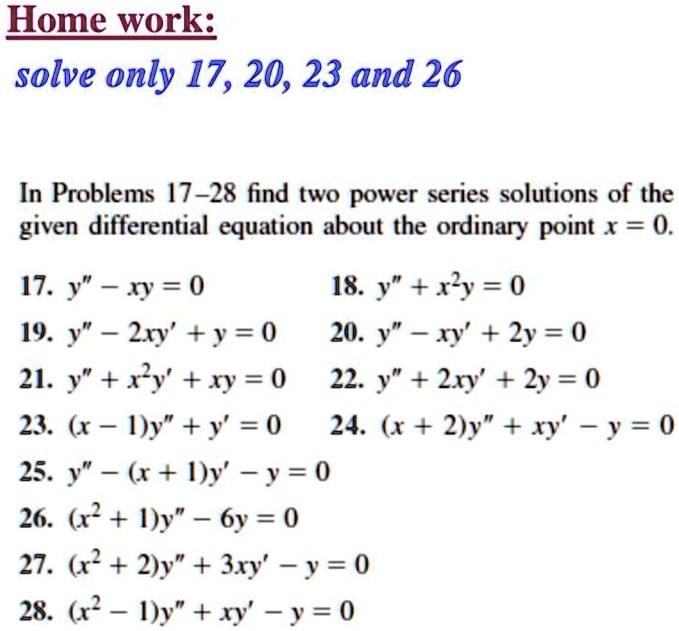「(1-x^2)y''-2xy'+2y=0 power series」の画像ギャラリー、詳細は各画像をクリックしてください。

Please help Thanks in advance We have x2=2xy 3y2 = 0 Are there supposed to be 2 equal signs in this expression or is it x2Solve the following ordinary differential equations using the power series method (1x^2)y"2xy2y=0 Example solutions is in the picture Expert Solution Want to see the full answer?

Incoming Term: (1-x^2)y''-2xy'+2y=0 power series,